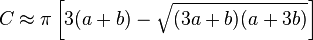Dec 27, 2008
Figurenschneider?
There was also a video (in German) showing off their work (h/t to Thistledown Puppets)
Dec 25, 2008
Meet Schubert!
Dec 19, 2008
How to Make a Puppet Pattern, Part II

 Look at that picture above again. See the dark curve marked C/2? That, you can see, will be the height of our pattern. That amount is simply half of the circumference, which means it is roughly the amount to the right: [4.5(a+b) - 1.5*sqrt((3a+b)(a+3b))]. That is by far the most difficult part of this process.
Look at that picture above again. See the dark curve marked C/2? That, you can see, will be the height of our pattern. That amount is simply half of the circumference, which means it is roughly the amount to the right: [4.5(a+b) - 1.5*sqrt((3a+b)(a+3b))]. That is by far the most difficult part of this process. The width of each piece will, like the sphere, be one-eighth (1/8) the circumference of the center circle. Remember that b is the radius of the center circle? That means the circumference is 2πb. Estimate that π is 3, and we get roughly 6b. Now divide that by eight, and we get .75b, as you can see in the formula to the left.
The width of each piece will, like the sphere, be one-eighth (1/8) the circumference of the center circle. Remember that b is the radius of the center circle? That means the circumference is 2πb. Estimate that π is 3, and we get roughly 6b. Now divide that by eight, and we get .75b, as you can see in the formula to the left. Now, you simply need to follow the instructions for the sphere. Choose your height-- A=2a, your width-- B=2b for the final product. Now, draw a vertical line equal to 4.5(a+b) - 1.5*sqrt((3a+b)(a+3b)). Through the midpoint of that line, draw a horizontal line equal to .75b. Now, copy the angle made by connecting the end of the horizontal line to the end of the vertical line (M), to make angle N. Make an isoceles triangle (as you can see to the right), and use its tip as a center for your compass to creat a curve connecting the top of the vertical line, the right end of the horizontal line, and the bottom of the vertical line.
Now, you simply need to follow the instructions for the sphere. Choose your height-- A=2a, your width-- B=2b for the final product. Now, draw a vertical line equal to 4.5(a+b) - 1.5*sqrt((3a+b)(a+3b)). Through the midpoint of that line, draw a horizontal line equal to .75b. Now, copy the angle made by connecting the end of the horizontal line to the end of the vertical line (M), to make angle N. Make an isoceles triangle (as you can see to the right), and use its tip as a center for your compass to creat a curve connecting the top of the vertical line, the right end of the horizontal line, and the bottom of the vertical line. 
 Just to demonstrate that the equation really isn't that hard, take my example from before. Say that I want my height to be 1.5 feet and my width to be 1 foot. Using the formula, we discover that the height is simply 2.27 feet and the width is .375 feet. I can now follow the rest of the instructions, cut out eight foam pieces, and creat my ellipsoid!
Just to demonstrate that the equation really isn't that hard, take my example from before. Say that I want my height to be 1.5 feet and my width to be 1 foot. Using the formula, we discover that the height is simply 2.27 feet and the width is .375 feet. I can now follow the rest of the instructions, cut out eight foam pieces, and creat my ellipsoid!Mathematical Caricatures?

Most caricatures are not done by mathematic means but by emphasizing already prominent features (while maintaining space relationships between different facial planes). What does that mean? Cartoonists are effectively using biological topology every day. Why do pictures that emphasize George Bush's hair not look like him while those that emphasize Rod Blagojevich's do? Why are Jimmy Carter's lips always big, while Richard Nixon's nose is always long? These are the identifying characteristics, and, I would posit, they are the planes that most strongly depart from the ideal face of beauty, be they bigger, smaller, wider, thinner, or whatever.
Dec 17, 2008
I was bored at work

Dec 15, 2008
ZooLights!

As much as I hate seeing the sun go down at 4:30pm during the winter, Christmas lights somehow make it a little more bearable. If you are in the Chicagoland area (and it's not frigid like today), be sure to take some time to see ZooLights at Lincoln Park Zoo. It's free, it's beautiful, and it provides a perfect melding of Christmastime and animals. I'm going this week sometime. Hope to see you there!
photo h/t stephanie says (via flickr)
Chicago Weather is Nuts
Chicago's warmest Christmas
Dear Tom,
When was Chicago's warmest Christmas?
Adam Bednarek, Dyer, Ind.
Dear Adam,
It was nearly 26 years ago in 1982 when Chicago experienced a Christmas that was warmer than most Easters. The afternoon temperature peaked at a balmy 64 degrees, twice the day's normal 32-degree high. While most Chicagoans celebrated a traditional holiday, some people were washing their cars, barbequing and even playing golf. Excited children got an unexpected chance to ride their new bikes and scooters outdoors. The very next year was payback time as the city shivered through its coldest Christmas. After a frigid Christmas Eve when the mercury dipped to minus 25, Christmas Day 1983 dawned with a low of 17 below and could only muster a high of minus 5. The back-to-back Christmases had an incredible temperature spread of 81 degrees, a true testament to Chicago's vigorous and volatile climate.
Obama Logos
This link is to the Obama logos that didn't make the cut. I especially like the speech-bubbles, though I understand why his final choice was, in many ways, ideal. The uniqueness of his name let the 'O' make his symbol very obvious (in a way that an 'M' likely wouldn't have been for McCain), and the center being a rising sun over stripes, which both evoked the American flag and the classic agrarian life (did anyone else notice that it looks like a freshly plowed field?), was, to put it bluntly, very impressive.
 That said, the Republican party needs to hire some people with eyes for aesthetics. Bold words won't cut it anymore. It didn't help either that McCain is old and white and his smiles are creepy. Even youthful supporters of his like me cringed a bit when watching him.
That said, the Republican party needs to hire some people with eyes for aesthetics. Bold words won't cut it anymore. It didn't help either that McCain is old and white and his smiles are creepy. Even youthful supporters of his like me cringed a bit when watching him.Also, the traditional Republican elephant is boring. It needs to be changed.

Dec 13, 2008
Making a Maquette
First, some reference points.
Here is the awesome site of Shane Zalvin, who has made some professional maquettes for Dreamworks productions (maquettes in the computer animating process are more conceptual, and 3-D character models are made off of them). He has some Disney sculptures as well, but they are for consumer products, not actual productions.
Here are two photos of Disney maquettes, one of Cookie from Atlantis and one of Koda and Kenai from Brother Bear.


For my yeti project, I've been working on the yeti himself as a maquette. Mine has not been baked (and likely won't be), though I'm looking at ways to make a mold for him so I can transfer the sculpture into resin.
How did the sculpture happen, though?
 First, I had to decide what type of clay I was going to use. I knew that I wanted a modeling clay of some type (since I don't have a kiln). I went to the Utrecht store in downtown Chicago and bought medium-pliability, gray-green Roma Plastina. I found that the stuff was perfect; easy to mold (after a little kneading), but firm enough to keep me from worrying that it would melt into itself on a hot day. It cuts pretty roughly, but with some work, it smooths very well and doesn't leave many fingerprints, which was an added bonus for me.
First, I had to decide what type of clay I was going to use. I knew that I wanted a modeling clay of some type (since I don't have a kiln). I went to the Utrecht store in downtown Chicago and bought medium-pliability, gray-green Roma Plastina. I found that the stuff was perfect; easy to mold (after a little kneading), but firm enough to keep me from worrying that it would melt into itself on a hot day. It cuts pretty roughly, but with some work, it smooths very well and doesn't leave many fingerprints, which was an added bonus for me.I already owned some cheaper, more oily modeling clay (I think I bought it at Hobby Lobby or Michael's or something). I used that to make a model for the head that I would eventually create out of the Roma Plastina. It was an easy way to try out what I wanted to do.
I came up with this head, and I really liked it. I made a head out of the Roma Plastina that was very similar to it, though more on that later.
 My first challenge was with the body. My yeti has a huge, heavy body, supported by relatively tiny legs. I needed some way to keep the body from being so heavy that it would make the legs collapse. My solution was aluminum foil. I molded it into the shape of the body and then covered it with a relatively thin (though far more than paper-thin) coat of clay.
My first challenge was with the body. My yeti has a huge, heavy body, supported by relatively tiny legs. I needed some way to keep the body from being so heavy that it would make the legs collapse. My solution was aluminum foil. I molded it into the shape of the body and then covered it with a relatively thin (though far more than paper-thin) coat of clay.I then began making the extremities. I used sculpting wire around each arm and leg, sticking it into the foil, to make them keep their shape and to keep them better attached to the body. When possible, I wanted to avoid having the clay supporting itself.
I also made a base (which was basically a messy-looking cut of the clay--it looked like rock).
Dec 12, 2008
I Heart My Family
Slate Goes Reenactor!
Once humans spent most of their days doing useful things with their hands, and I realized that we were designed to get a deep satisfaction from this. As Hughes put it, "You have the feeling people were supposed to do this kind of work, rather than data entry, which is amazingly horrible."
An article today on Slate magazine about Emily Yoffe's 'brief, inspiring career as a historical re-enactor'. In it, she discusses her time portraying a colonial farm woman at the Claude Moore Colonial Farm in McLean, Virginia. The place is apparently right across the street from the CIA headquarters in Langley, Virginia. How weird. I love the strange juxtapositions that inevitably arise when reenacting.
It's cool to hear someone else discuss it. One of the best parts of recreating the past is when you get the 'high'--the feeling that you really are where you up to then had only been pretending to be. She got it through the mundane tasks of growing tobacco and dealing with livestock.
Reenacting helps you remember that there was a time, not so far back, when people lived with a purpose that was more than waiting for the weekend. I had a class a few years ago about American demographics in marketing. Robert Fogel taught it, and he showed us how the average American in 1800 only had about 30 minutes of free time each day, whereas the average American today has around eight hours. That's amazing when you think about it. Additionally, 50% of a typical family income was used to pay for food. Today, it hovers around 10%. Disposable income for the masses is a relatively recent phenomenon. People in the past worked hard, not to make a living, but to survive.
Long and short of it, historical reenacting is fun and educational--practically and emotionally--and everyone should try it out at some point.
Shameless Plug for Awesome Toy
Introducing Spuda Fett:

Someone at Disney or Lucasfilm had way too much time on his/her hands.
Buy him here.
Photo credit: Bratislabat via Flickr
Dec 9, 2008
How to Make a Puppet Pattern, Part 1
If you don't want to read the process, just go to the end of the post, and you will find simple directions on how to make a pattern.
Sphere

Take a look at my handy picture. You will see that in a sphere the distance from the center to the surface on all three axes (X, Y, Z or length, width, and height) is the same. I've labelled it R (for 'radius', since I'm clever). R will make our calculations very convenient, as you will see if you make it to the spheroids.

When we make a sphere out of foam, we will need to focus on the surface. (since it will be hollow). We will cut it into kayak-shaped pieces, just like the longitude lines on a globe (see picture to the left). I will use eight pieces (though you can easily to more, and less easily do less; I wouldn't recommend less than five or six).
How will we figure out the measurements for these pieces?
First, consider how tall you want to make your sphere. How big will the puppet's head or body be? That length is the length from the top to the bottom of the sphere, which, as you can easily see, is 2R. This allows you to figure out R (by dividing the length you want by 2).
 Now, have you ever noticed that if you cut a sphere in half along any of the axes (X,Y, or Z), the circle that you get is the exact same size? That's important here. We're going to cut the sphere along the X axis (with an X/Z plane). We get a circle (which I've highlighted in blue). You can see that the height of our sphere piece will be half the circumference of that circle.
Now, have you ever noticed that if you cut a sphere in half along any of the axes (X,Y, or Z), the circle that you get is the exact same size? That's important here. We're going to cut the sphere along the X axis (with an X/Z plane). We get a circle (which I've highlighted in blue). You can see that the height of our sphere piece will be half the circumference of that circle. That's handy for us. The circle has a radius of R, which means that it has a circumference of 2πR (see here for that formula). We want half of that, which is πR. This will be the height of our piece. I have drawn it out in the picture to the left.
That's handy for us. The circle has a radius of R, which means that it has a circumference of 2πR (see here for that formula). We want half of that, which is πR. This will be the height of our piece. I have drawn it out in the picture to the left. What will the width be, you ask? Great question! I am making this out of eight pieces, which means that the width of each piece should be one-eighth (1/8) of the entire length of the sphere's equator. Note that if you are making it out of more or fewer pieces, the fraction will reflect the number of pieces you are making (so, ten pieces, 1/10; four pieces, 1/4). Because this is a sphere, once again, the length of the sphere's equator is equal to 2πR, which means that the length of our piece will be 2πR/8 = πR/4. This is important. It means that the width of our pattern will be one-fourth (1/4) the height. Since a sphere is symmetric in all directions when cut through the center, the width and the height will bisect each other. I've drawn it out to the left. Oh, and don't worry about π right now. We're just going to estimate it as 3 at the end.
What will the width be, you ask? Great question! I am making this out of eight pieces, which means that the width of each piece should be one-eighth (1/8) of the entire length of the sphere's equator. Note that if you are making it out of more or fewer pieces, the fraction will reflect the number of pieces you are making (so, ten pieces, 1/10; four pieces, 1/4). Because this is a sphere, once again, the length of the sphere's equator is equal to 2πR, which means that the length of our piece will be 2πR/8 = πR/4. This is important. It means that the width of our pattern will be one-fourth (1/4) the height. Since a sphere is symmetric in all directions when cut through the center, the width and the height will bisect each other. I've drawn it out to the left. Oh, and don't worry about π right now. We're just going to estimate it as 3 at the end.
Well, this is great. We have the height and the width. we have just one more problem. The sides must be curved, since the sphere itself is curved on its surface. We need to figure out how to connect the endpoints of the width and the height. See figure to the left.
 To find this curve is surprisingly simple. We need a circle that has a center on the same axis as our width (the short line). The distance from that center to the end of our width line (the radius of that circle) must be the same as distance from the center of that circle to the top and the bottom of the height line. How can we do this? We need an isoceles triangle, with the two equal sides going from the far end of our width line and from the top of the height line to the center of that circle. Using a compass and straightedge, it's not too hard. Just copy angle M (see picture) to become angle N.
To find this curve is surprisingly simple. We need a circle that has a center on the same axis as our width (the short line). The distance from that center to the end of our width line (the radius of that circle) must be the same as distance from the center of that circle to the top and the bottom of the height line. How can we do this? We need an isoceles triangle, with the two equal sides going from the far end of our width line and from the top of the height line to the center of that circle. Using a compass and straightedge, it's not too hard. Just copy angle M (see picture) to become angle N.
Now, using the compass, make a curve from the bottom of the height line to the top of the height line. Copy that to the other side, and you have your pattern. Make eight of these out of sheet foam, and, presto! you have a sphere.

Check in soon to see how to make a spheroid.